1 Chalkboard

Figure 1: Connected region in the plane
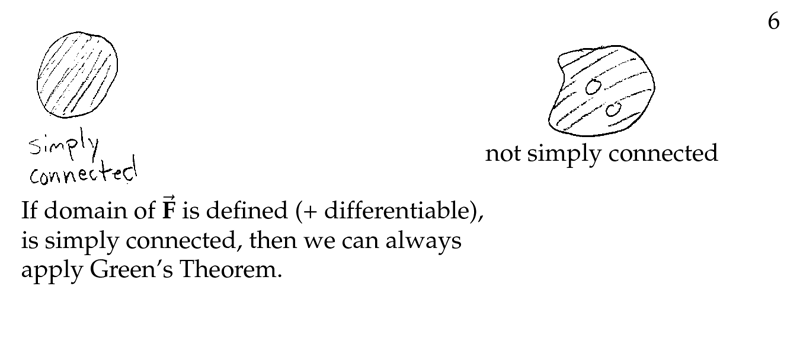
Figure 2: Where can I apply Green’s Theorem?

Figure 3: Correct definition of \(\vb{F}\) conservative
2 What is a simply-connected region?
2.1 Front
What is a simply-connected region?
Two dimensional region \(D\)
2.2 Back
Whenever a simple closed curve \(C\) lies entirely in \(D\), then its interior also lies entirely in \(D\)
Examples:
- \(xy\text{-plane}\)
- the right-half plane where \(x \geq 0\)
- the unit circle
Regions without “holes”
3 Can I apply Green Theorem directly in a simply-connected region?
3.1 Front
Can I apply Green Theorem directly in a simply-connected region?
3.2 Back
Yes, you can
4 Can I say that \(\vb{F}\) is conservative if…
4.1 Front
Can I say that $\vb{F}$ is conservative if…
\(\vb{F}\) is continuously differentiable in a simply-connected region \(D\), and \(\text{curl} \vb{F} = 0\)
4.2 Back
Yes, \(\vb{F} = \grad{f}\) for some \(f(x,y)\) and if \(\vb{F} = \ev{M,N}\), then \(M_y = N_x\)
5 Is an exact differential?
5.1 Front
Is an exact differential?
\({\displaystyle \frac{y \dd{x} - x \dd{y}}{y^2}}\)
5.2 Back
Where \(\vb{F} = \ev{M,N}\) are continuously differentiable wherever \(y \neq 0\), for example, two half-planes above and below \(x\text{-axis}\). And these are both simply-connected.
- \(M = \frac{1}{y}\)
- \(N = \frac{-x}{y^2}\)
As \(M_y = N_x = -1/y^2\), in each half-plane the differential is exact
6 For this vector field
6.1 Front
For this vector field
Find the domain on which it is defined and continuously differentiable. Is that domain simply connected?
\(\vb{F} = \abs{x} \vu{i} + 0 \vu{j}\)
6.2 Back
Is not differentiable when \(x=0\), the region is the union of the open left and right half-planes and is not simply connected
7 Can we use the FTC for line integrals for compute flux of \(\vb{F}\)
7.1 Front
Can we use the FTC for line integrals for compute flux of $\vb{F}$
7.2 Back
Can we use the FTC for line integrals for compute flux of \(\vb{F}\)
No, only it’s valid for line integrals of work of \(\vb{F}\)